Welcome to The STORM Count, our new article series analyzing the mathematics of our favorite Storm deck. While I will be including some of the theoretical concepts behind the math that we use, the goal is that the information presented in this article is accessible for as many of our readers as possible.
Today, we are going to look at the exact numbers behind one of the biggest strengths of The EPIC Storm (TES), especially when compared to Ad Nauseam Tendrils (ANT): the card Ad Nauseam itself!
The Math
The expected value of a random event is analogous to a mean, also known as an average. It has a wide range of uses such as determining the fairness of a game of chance. The expected value is a way of saying how much a particular trial of a random event is worth on average. For example, let's say you were to play a game where you pay $1 to roll a standard six-sided die, you win $12 if you roll a 6, and you win $0 for any other roll. The expected value of this game is $1. This means that, on average, you should gain $1 every time you play this game. It's important to notice that a gain of exactly $1 is impossible. Instead, think about what it looks like if you play the game six times and get each equally-likely outcome exactly once. On five of those games, you would lose $1. On the sixth, you would lose $1 but gain $12. Altogether, you would have lost $6 and gained $12 across six games, an average gain of $1 per game. That is how expected value works.
The Law of Large Numbers: As the number of trials approaches infinity, the sample average of all trials converges to the expected value.
The Gambler's Fallacy: If an event occurs less often than expected, it will be more likely to occur in the future. (This is a fallacy and is false)
The Law of Large Numbers (LLN) is important to our understanding of random events and how they occur. The most important part is that the LLN only guarantees that the observed average and the expected value will approach each other as the number of trials approaches infinity. That's a lot of trials. In our die-game example, you could easily play over a hundred times and still lose money. This is where The Gambler's Fallacy tends to rear its ugly head. If you know that the expected value of the game is positive and you've lost money over the last one hundred games, it is very easy to think that you must be more likely to start winning money soon. This is absolutely not the case. Rolls of a six-sided die are independent, meaning the result of one roll does not affect the outcome of the next roll. Every time, the probability of winning the $12 is exactly 1 in 6. That never changes.
The Magic
The EPIC Storm
Main Deck
- 4 Burning Wish
- 4 Infernal Tutor
- 4 Brainstorm
- 4 Ponder
- 4 Thoughtseize
- 2 Duress
- 1 Cabal Therapy
- 1 Chain of Vapor
- 1 Empty the Warrens
- 1 Ad Nauseam
Sideboard
Ad Nauseam Tendrils
Main Deck
- 4 Infernal Tutor
- 4 Brainstorm
- 4 Ponder
- 4 Preordain
- 4 Thoughtseize
- 3 Duress
- 2 Past in Flames
- 1 Tendrils of Agony
- 1 Dark Petition
- 1 Ad Nauseam
Sideboard
The EPIC Storm
Main Deck
- 4 Burning Wish
- 4 Infernal Tutor
- 4 Brainstorm
- 4 Ponder
- 4 Thoughtseize
- 2 Duress
- 1 Cabal Therapy
- 1 Chain of Vapor
- 1 Empty the Warrens
- 1 Ad Nauseam
Sideboard
Main Deck
- 4 Burning Wish
- 4 Infernal Tutor
- 4 Brainstorm
- 4 Ponder
- 4 Thoughtseize
- 2 Duress
- 1 Cabal Therapy
- 1 Chain of Vapor
- 1 Empty the Warrens
- 1 Ad Nauseam
Sideboard
Ad Nauseam Tendrils
Main Deck
- 4 Infernal Tutor
- 4 Brainstorm
- 4 Ponder
- 4 Preordain
- 4 Thoughtseize
- 3 Duress
- 2 Past in Flames
- 1 Tendrils of Agony
- 1 Dark Petition
- 1 Ad Nauseam
Sideboard
Main Deck
- 4 Infernal Tutor
- 4 Brainstorm
- 4 Ponder
- 4 Preordain
- 4 Thoughtseize
- 3 Duress
- 2 Past in Flames
- 1 Tendrils of Agony
- 1 Dark Petition
- 1 Ad Nauseam
Sideboard
These are the decklists we will use for all of our calculations in this article.
Theorem: The expected value of the number of cards drawn with Ad Nauseam in The EPIC Storm is greater than in Ad Nauseam Tendrils.
This is a fairly easy theorem to prove in the most abstract of cases. First, we find the average converted mana cost of the non-Ad Nauseam cards in each deck.
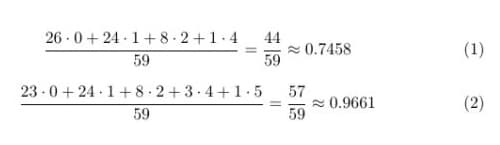
This demonstrates that the average converted mana cost of the cards in TES is less than that of ANT. Consequently, a TES pilot will be able to draw more cards on average than a player on ANT. How many cards, exactly?

Now, the number of games where you will cast Ad Nauseam with all 59 other cards in your library is approximately zero. This means that the utility of these specific numbers is quite limited. However, they do give us a baseline for understanding how converted mana costs in each deck affect the card-draw potential for Ad Nauseam. Let's put together a reasonable scenario for each deck so we can see numbers in the wild.
TES Scenario
Opening hand (on the play): Polluted Delta, Duress, Thoughtseize, Thoughtseize, Brainstorm, Dark Ritual, Lotus Petal
Turn 1: Play Polluted Delta, fetch an Underground Sea, cast Thoughtseize (Plains, Rishadan Port, Thalia, Guardian of Thraben, Mother of Runes, Stoneforge Mystic, Sword of Fire and Ice, Flickerwisp). Take Thalia, Guardian of Thraben. Opponent draws, plays Plains, and casts AEther Vial.
Turn 2: Draw Bloodstained Mire. Play Bloodstained Mire, cast Brainstorm. Draw Infernal Tutor, Lion's Eye Diamond, Ponder. Put back Ponder and Thoughtseize. Fetch a Badlands. Cast Lotus Petal, Lion's Eye Diamond, Dark Ritual, and Infernal Tutor, sacrificing the Lion's Eye Diamond for BBB. Get Ad Nauseam and cast it (Storm count 6, no mana floating).
Summary: Four lands, two zero-mana artifacts, four one-mana spells (it was six at one point, but we put two back with Brainstorm), one two-mana spell, and Ad Nauseam are all absent from our deck. Also, we have paid four life among two fetchlands and one Thoughtseize. We can now calculate how many cards we can expect to draw.
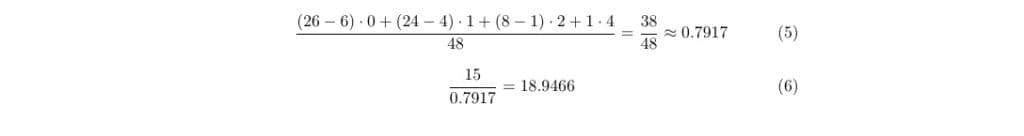
Equation (6) shows how many cards we can expect to draw with our life total.
We can see from Equation 5 that our average converted mana cost has increased from Equation 1 above. Also, the higher average converted mana cost combined with our lower life total means that the expected value of our card draw is fewer by more than six cards (compare Equation 6 to Equation 3).
ANT Scenario
Opening hand (on the play): Misty Rainforest, Duress, Ponder, Preordain, Lotus Petal, Infernal Tutor, Past in Flames.
Turn 1: Play Misty Rainforest, fetch Underground Sea, cast Duress. (Ancient Tomb, City of Traitors, Reality Smasher, Reality Smasher, Thought-Knot Seer, Chalice of the Void, Trinisphere). Take Chalice of the Void. Opponent draws, plays Ancient Tomb, and passes.
Turn 2: Draw Tropical Island. Play it, cast Preordain. Scry Tendrils of Agony to bottom, draw Thoughtseize. Cast Thoughtseize. (City of Traitors, Reality Smasher, Reality Smasher, Thought-Knot Seer, Thought-Knot Seer, Trinisphere). Take Trinisphere. Opponent draws, plays City of Traitors, casts Thought-Knot Seer, and exiles Infernal Tutor.
Turn 3: Draw Brainstorm, cast it. Draw Dark Ritual, Infernal Tutor, and Lion's Eye Diamond. Put back Ponder and Past in Flames. Cast Lotus Petal, Lion's Eye Diamond, Dark Ritual, and Infernal Tutor, sacrificing the Lion's Eye Diamond for BBB. Get Ad Nauseam and cast it (Storm count 6, no mana floating).
Summary: Three lands, two zero-mana artifacts, five one-mana spells (it was six at one point, but we put one back with Brainstorm), two two-mana spells, and Ad Nauseam are all absent from our deck. Between the Misty Rainforest and Thoughtseize, we have paid three life. We can now calculate how many cards we can expect to draw.
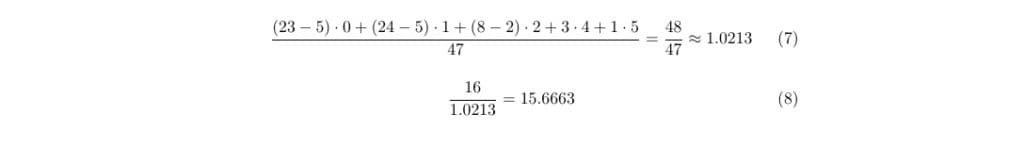
Equation (8) shows how many cards we can expect to draw with our life total.
The Lesson
While this is a simplified view of the situation, the math confirms that The EPIC Storm is able to regularly draw more cards than Ad Nauseam Tendrils. The inclusion of Chrome Mox in TES plus Past in Flames and Dark Petition in ANT serve to decrease the average CMC of TES and increase it for ANT. Thus, for similar life totals, TES will see more cards from Ad Nauseam. This is why we frequently refer to The EPIC Storm as "the better Ad Nauseam deck" in discussions. What this math does not show is how many cards you would need to see in order to win the game on the spot. That is much more complicated because there are many paths to victory from a resolved Ad Nauseam.
An important takeaway from this analysis is this: whenever you are considering altering a decklist, make sure to look at how this will affect the average converted mana cost of your deck. In general, adding a card whose CMC is above the average will increase the average, and adding a card below the average will decrease it. The inverse is true for removing cards. So, for example, if you swap a one-mana Rite of Flame for a zero-mana Mox Opal, you decrease the average and make your Ad Nauseams better. If you swap a zero-mana Chrome Mox for a two-mana Cabal Ritual, you increase the average and make your Ad Nauseams worse. Ad Nauseam is one of the single most-powerful cards in the deck and its influence on deckbuilding should be respected.
Thank you for reading! I hope this article gave you some insight into the mathematics of Ad Nauseam and how it plays out differently in TES and ANT. Please drop me a line if you have any other math subjects you would like to hear about! Until next time, may your Storm counts always be lethal.










